
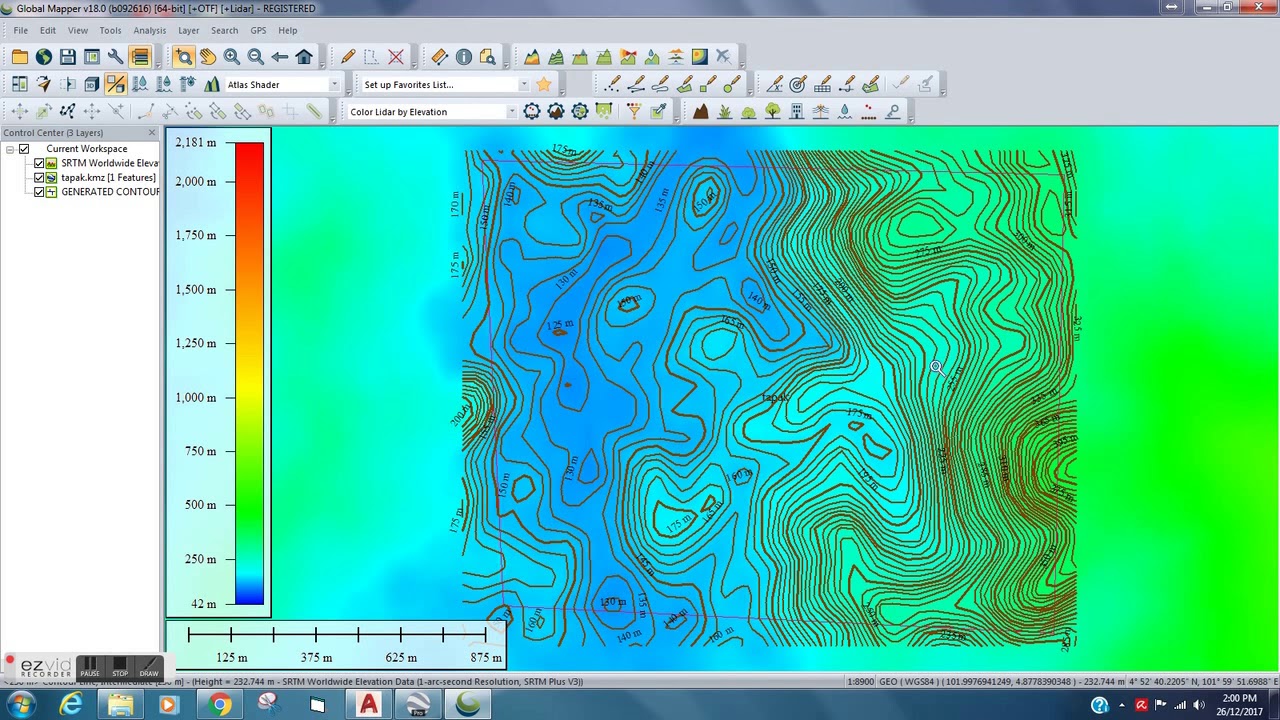
This slice is the intersection of the graph with the plane $z=c$. The key point is that a level curve $f(x,y)=c$ can be thought of as a horizontal slice of the graph at height $z=c$. The below graph illustrates the relationship between the level curves and the graph of the function. In the level curve plot of $f(x,y)$ shown below, the smallest ellipse (If $c$ is negative, then both denominators are positive.) ForĮxample, if $c=-1$, the level curve is the graph of $x^2 + 2y^2=1$. Rewrite the equation for the level curve as As long as $c<0$, this graph is an ellipse, as one can For someĬonstant $c$, the level curve $f(x,y)=c$ is the graph of We return to the above example function $f(x,y) = -x^2-2y^2$. Together in a level curve plot, which is sometimes called a contour We can plot the level curves for a bunch of different constants $c$ A function has many level curves, as one obtains aĭifferent level curve for each value of $c$ in the range of $f(x,y)$. A level curve is simply aĬross section of the graph of $z=f(x,y)$ taken at a constant value, Of two variables into a two-dimensional plot is through level curves.Ī level curve of a function $f(x,y)$ is the curve of points $(x,y)$ One way to collapse the graph of a scalar-valued function The nice part of of level sets is that they live in the same dimensions as the domain of the function.Ī level set of a function of two variables $f(x,y)$ is a curve in the two-dimensional $xy$-plane, called a level curve.Ī level set of a function of three variables $f(x,y,z)$ is a surface in three-dimensional space, called a level surface. Moreover, the graph of a function $f(x,y,z)$ of three variables would be the set of points $(x,y,z,f(x,y,z))$ in four dimensions,Īnd it would be difficult to imagine what such a graph would look like.Īnother way of visualizing a function is through level sets, i.e., the set of points in the domain of a function where the function is constant. To draw and visualize than two-dimensional plots. Three-dimensional plots, such as the above figure, are more difficult A graph of the function $f(x,y)=-x^2-2y^2$ over the domain $-2 \le x \le 2$ and $-2 \le y \le 2$.


 0 kommentar(er)
0 kommentar(er)
